0, one has that 6 is 6 stable, and since f'(10) < 0,="" one="" has="" that="" 10="" is="" unstable.="" in="" the="" long="" term="" there="" will="" be="" 6="" zombies="" in="" my="" lab.="" biologically="" meaningful="" steady="" states="" are="" z1="6" and="" z2="10." since="" f'(6)=""> 0, one has that 6 is unstable, and since f'(10) < 0,="" one="" has="" that="" 10="" is="" stable.="" in="" the="" long="" term="" there="" will="" be="" 10="" "/="">
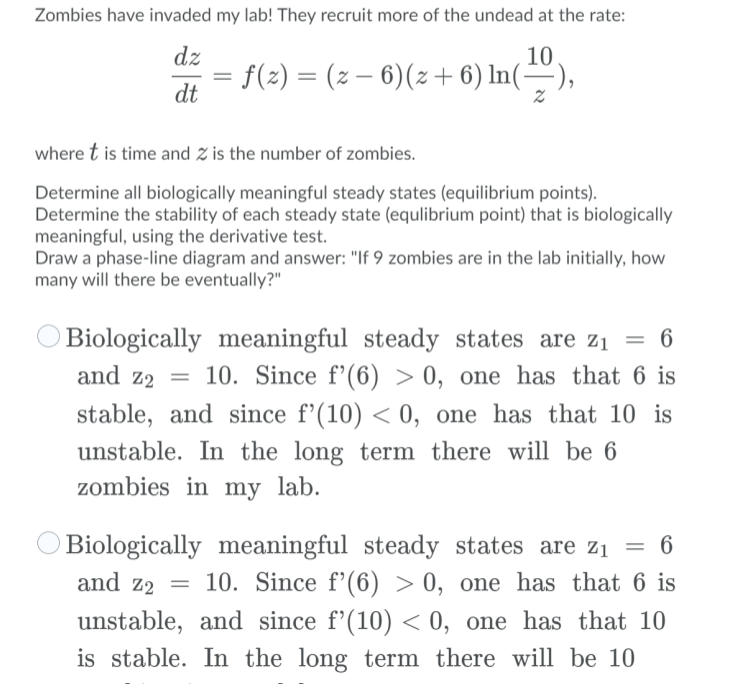
Extracted text: Zombies have invaded my lab! They recruit more of the undead at the rate: dz = f(2) = (z – 6)(z + 6) In(÷), 10 dt where t is time and Z is the number of zombies. Determine all biologically meaningful steady states (equilibrium points). Determine the stability of each steady state (equlibrium point) that is biologically meaningful, using the derivative test. Draw a phase-line diagram and answer: "If 9 zombies are in the lab initially, how many will there be eventually?" Biologically meaningful steady states are z1 and z2 = 10. Since f'(6) > 0, one has that 6 is 6 stable, and since f'(10) < 0,="" one="" has="" that="" 10="" is="" unstable.="" in="" the="" long="" term="" there="" will="" be="" 6="" zombies="" in="" my="" lab.="" biologically="" meaningful="" steady="" states="" are="" z1="6" and="" z2="10." since="" f'(6)=""> 0, one has that 6 is unstable, and since f'(10) < 0,="" one="" has="" that="" 10="" is="" stable.="" in="" the="" long="" term="" there="" will="" be="">
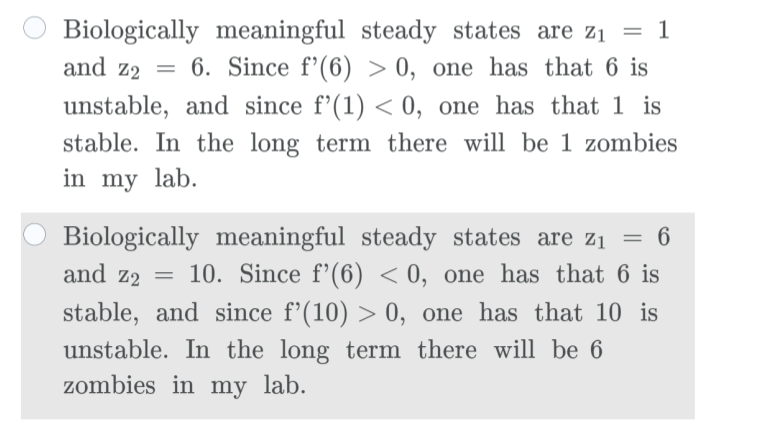
Extracted text: Biologically meaningful steady states are z1 = and z2 6. Since f'(6) > 0, one has that 6 is unstable, and since f'(1) < 0,="" one="" has="" that="" 1="" is="" stable.="" in="" the="" long="" term="" there="" will="" be="" 1="" zombies="" in="" my="" lab.="" biologically="" meaningful="" steady="" states="" are="" z1="6" and="" z2="10." since="" f'(6)="">< 0,="" one="" has="" that="" 6="" is="" stable,="" and="" since="" f'(10)=""> 0, one has that 10 is unstable. In the long term there will be 6 zombies in my lab.