Please solve correctly in 20 minutes fill Al the boxes and get the thumbs up
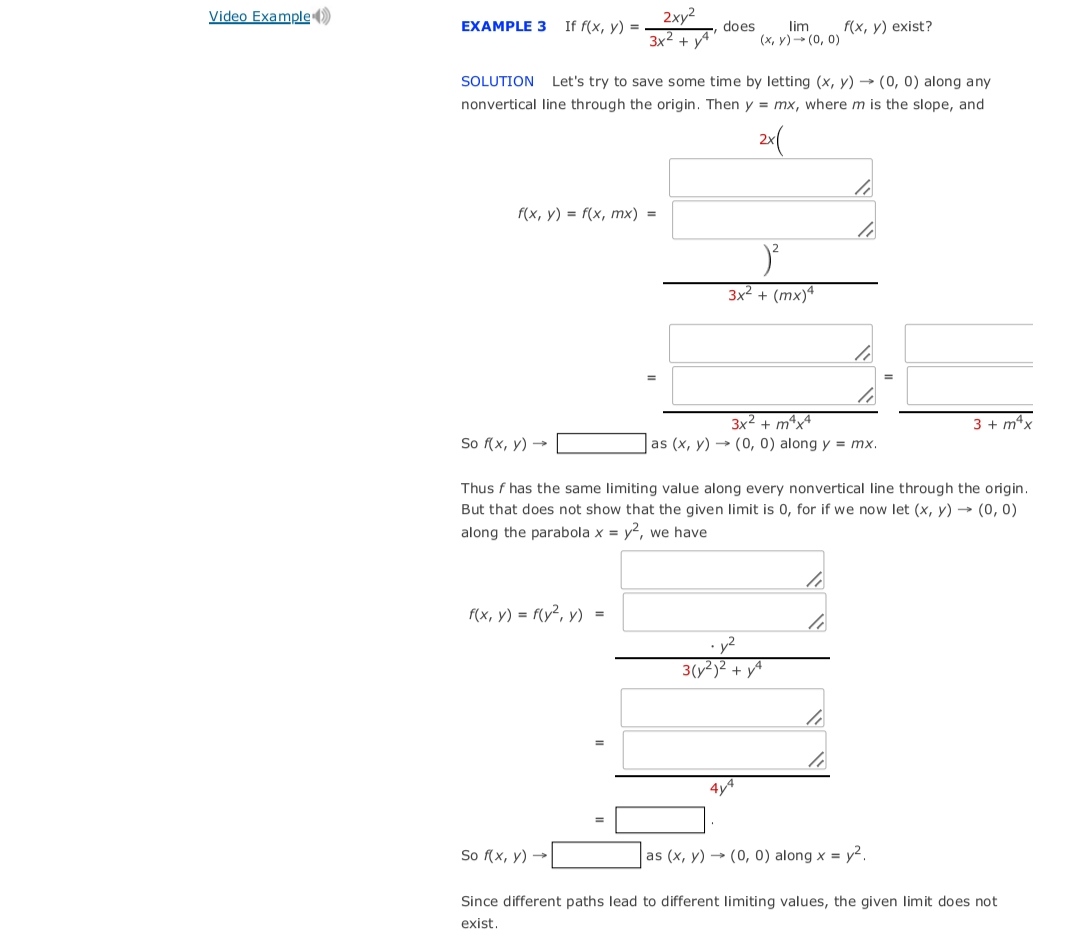
Extracted text: Video Example ) 2xy2 3x2 + yA EXAMPLE 3 If f(x, y) = does lim (x, y)- (0, 0) f(x, y) exist? SOLUTION Let's try to save some time by letting (x, y) → (0, 0) along any nonvertical line through the origin. Then y = mx, where m is the slope, and f(x, у) %3D f(x, тх) %3 3x² + (mx)ª 3x2 + mªx4 3 + m*x So f(x, y) → as (x, y) → (0, 0) along y = mx. Thus f has the same limiting value along every nonvertical line through the origin. But that does not show that the given limit is 0, for if we now let (x, y) → (0, 0) along the parabola x = y?, we have f(x, y) = f(y², y) = 3(y²)² + yA 4y4 So f(x, y) → as (x, y) → (0, 0) along x = y². Since different paths lead to different limiting values, the given limit does not exist.