I need help with these two questions?

Extracted text: The quality department at an electronics company has noted that, historically, 92% of the units of a specific product pass a test operation, 6% fail the test but are able to be repaired, and 2% fail the test and need to be scrapped. Due to recent process improvements, the quality department would like to test if the rates have changed. A recent sample of 500 parts revealed that 475 parts passed the test, 18 parts failed the test but were repairable, and 7 parts failed the test and were scrapped. (You may find it useful to reference the appropriate table: chi-square table or F table) a. Choose the appropriate alternative hypothesis for the test. At least one of the p; (i= 1, 2, 3) differs from its hypothesized value. O All p; (i= 1, 2, 3) values differ from its hypothesized value. b-1. Compute the value of the test statistic. (Round the intermediate calculations to at least 4 decimal places and final answer to 3 decimal places.) Test statistic
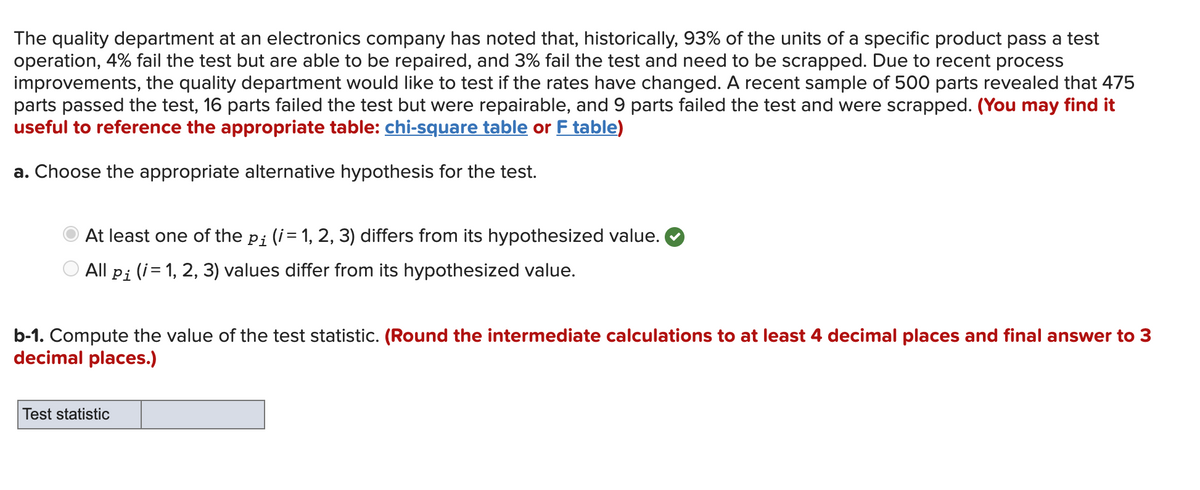
Extracted text: The quality department at an electronics company has noted that, historically, 93% of the units of a specific product pass a test operation, 4% fail the test but are able to be repaired, and 3% fail the test and need to be scrapped. Due to recent process improvements, the quality department would like to test if the rates have changed. A recent sample of 500 parts revealed that 475 parts passed the test, 16 parts failed the test but were repairable, and 9 parts failed the test and were scrapped. (You may find it useful to reference the appropriate table: chi-square table or F table) a. Choose the appropriate alternative hypothesis for the test. At least one of the pi (i= 1, 2, 3) differs from its hypothesized value. O All pi (i= 1, 2, 3) values differ from its hypothesized value. b-1. Compute the value of the test statistic. (Round the intermediate calculations to at least 4 decimal places and final answer to 3 decimal places.) Test statistic