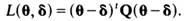Suppose we restrict consideration to the class of unbiased estimators for 8 (i.e., estimators for which
Give a decision-theoretic formulation of the "minimum variance unbiased estimator" criterion.
In Example 4, verify that
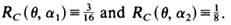
Consider the regression setup where
b = (b1
... , bp)' being a vector of regressor variables,
being a vector of unknown regression coefficients, and
being a
random error (u2
known, for simplicity). Some data, X, is available to estimate
denote the estimator. The goal of the investigation, however, is to predict future (independent) values of Y arising from this model. Indeed, such Y will be predicted (for each corresponding b) by
and the loss in estimating Y by
is squared-error prediction loss,
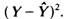
(a) Show, for a given b, that choice of .3(x) in the prediction problem is equivalent to choice of 3(x) in the problem of estimating 0 under loss
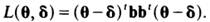
(b) Suppose
(independent of future Yand past X). Show that the prediction problem is equivalent to the problem of estimating 0 under loss
