Suppose that you are in the business of drilling a tiny hole in the exact center of a square piece of wood. In the past, the holes you have drilled were, on average, in the center of the wood, and the x- and y-coordinates each had a standard deviation of 0.1 inch. Also, the drilling process has been stable—that is, the holes average being in the center of the square, and the deviations from the center of the square (measured in both the x- and y-coordinates) follow a normal distribution with mean 0 and standard deviation 0.1 inch. This means, for example, that the x-coordinate is within 0.1 inch of the center for 68% of the holes, the xcoordinate is within 0.2 inch of the center for 95% of the holes, and the x-coordinate is within 0.3 inch of the center for 99.7% of the holes. This describes the inherent variability in the drilling process. Unless you change the hole-drilling process, you must accept this amount of variation.
Now suppose that you drill a hole and its x- and y-coordinates are x =- 0.1 and y = 0 [where the center of the square has coordinates (0, 0)]. A natural reaction is to reduce the x-setting of the drill by 0.1 to correct for the fact that the x-coordinate was too high. Then if the next hole has coordinates x =-0.2 and y =0.1, you might try to increase the x-coordinate by 0.2 and decrease the y-coordinate by 0.1. Deming’s funnel experiment shows that this method of continually readjusting a stable process—he calls it “tampering”— actually increases the variability of the coordinates of the position where the hole is drilled. In other words, tampering generally make a process worse! To illustrate the effects of tampering, Deming placed a funnel above a target on the floor and dropped small balls through the funnel in an attempt to hit the target. As he demonstrated, many balls did not hit the target. His goal, therefore, was to make the balls fall as close to the target as possible. Deming proposed four rules for adjusting the positioning of the funnel.
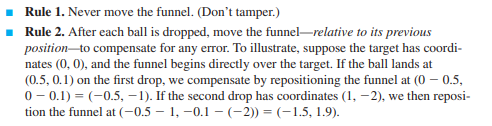
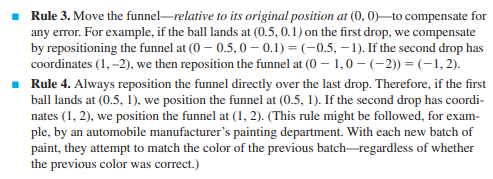
Do you believe any of these latter three rules outperform rule 1, the “leave it alone” rule? Is so, read on—you might be surprised.
Objective To use simulation to see the effect of tampering with a stable process, as opposed to leaving it alone.