Suppose that the data set contains three variables, and the normalized versions are labeled as
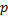
1,
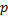
2, and
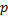
3. Also, assume that the PCA assumption is
p
=

1
v
1
+ α2
v
2, where
v
1
= (
11,

21,

31)
T
and
v
2
= (
12,

22,

32)
T
are the first two columns in the matrix
V.
(a) Show that the PCA assumption can be written as
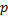
3
=

1
+
2, where
 = (
= (
31

22
−

32

12)/ = (
= (
32

11
−

31

21)/ , and
, and
 =
=

11

22
−

12

21. This assumes, of course, that
 0.
0.
(b) Using the result from part (a), show that the PCA assumption, when expressed in terms of the original, unnormalized, data variables, can be written as

where

1,

2
and

3
are the means of

1,

2
and

3, respectively. Also,
 =
=

3/
1
and
 =
=

3/
2, where

1,

2, and

3
are the scale factors used for the respective variables.