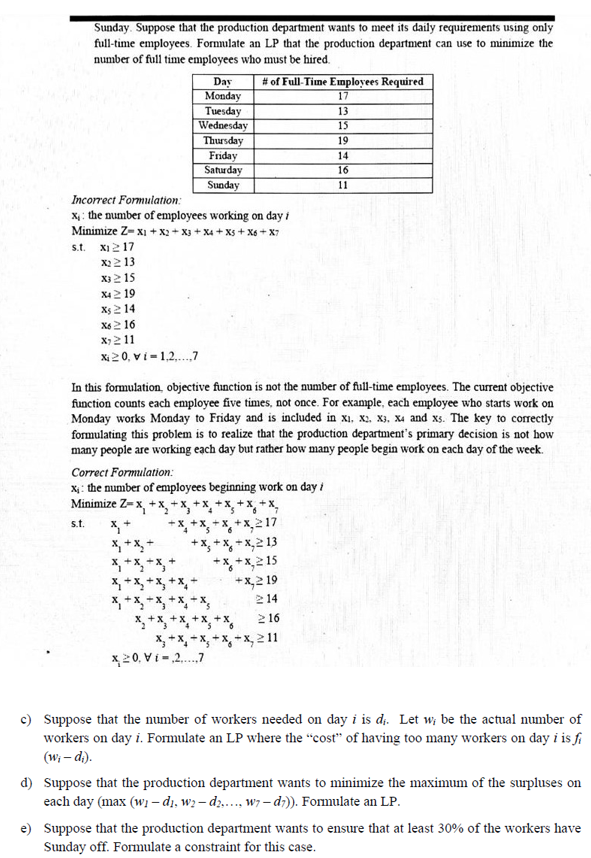
Extracted text: Sunday. Suppose that the production department wants to meet its daily requirements using only full-time employees. Formulate an LP that the production department can use to minimize the number of full time employees who must be hired. Day Monday Tuesday Wednesday # of Full-Time Employees Required 17 13 15 Thursday Friday Saturday Sunday 19 14 16 11 Incorrect Formulation: X, : the number of employees working on day i Minimize Z- xI + x2 + X3 + X4 + Xs + X6 + X7 s.t. XI2 17 x2 2 13 X) 215 X42 19 Xs 2 14 X6 2 16 X)211 Xi 20, vi - 1,2..7 In this formulation, objective function is not the number of full-time employees. The current objective function counts each employee five times, not once. For example, each employee who starts work on Monday works Monday to Friday and is included in x1, x2. X3, x4 and xs. The key to correctly formulating this problem is to realize that the production department's primary decision is not how many people are working each day but rather how many people begin work on each day of the week. Correct Formulation: x: : the number of employees beginning work on day i x,+x, +x, *, + x,217 +x, + x, + x,2 13 *+x,2 15 *X,2 19 214 Minimize Z- x, +x,** x, + X, + X, + s.t. X, + X, + x, + x, + *, +x, *x, +x, + X, X, +x, +x, + X, +X, X, +x, + x, + X, + x, 211 x2 0, V i-2.7 2 16 c) Suppose that the number of workers needed on day i is d.. Let w, be the actual number of workers on day i. Formulate an LP where the “cost" of having too many workers on day i is fi (W; – di). d) Suppose that the production department wants to minimize the maximum of the surpluses on each day (max (w1 – dı, w2 – dz.., w7 –d;)). Formulate an LP. e) Suppose that the production department wants to ensure that at least 30% of the workers have Sunday off. Formulate a constraint for this case.