Shows NRMSEs for various estimators of the innovation variance discussed in Section 8.7. The left-hand plot of that figure shows results based upon 10,000 realizations from the AR(2) process of Equation (34), and the right-hand plot, from the AR(4) process of Equation (35a). Consider now the AR(2) processes
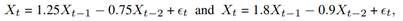
where, in both cases,
 is a white noise process with unit variance (these are used as examples in Pukkila and Nyquist, 1985, and Walden, 1995).
is a white noise process with unit variance (these are used as examples in Pukkila and Nyquist, 1985, and Walden, 1995).
(a) Compute the SDFs for both processes, and plot them on a decibel scale versus frequency. Use these plots to determine the dynamic ranges of these SDFs as defined by Equation (177a) for comparison with those for the AR(2) process of Equation (34) (14 dB) and the AR(4) process of Equation (35a) (65 dB). (C&E [5] for Section 9.2 discusses computation of AR SDFs.)
(b) For each of the two AR(2) process of interest here, create 10,000 realizations of sample size N = 1024 using the procedure described in Section 11.1. For each process, use these realizations to compute innovation variance estimates based upon the estimator
 of Equation (404c); the estimator
of Equation (404c); the estimator
 of Equation (404d) with L set to 2, 3, . . . , 25; the estimator
of Equation (404d) with L set to 2, 3, . . . , 25; the estimator
 of Equation (405a); and finally the estimator
of Equation (405a); and finally the estimator
 of Equation (405b) with K set to 2, 3, . . . , 25. For each of these estimates, compute NRMSEs analogous to that of Equation (406), and display the results in a manner similar to Comment upon your findings.
of Equation (405b) with K set to 2, 3, . . . , 25. For each of these estimates, compute NRMSEs analogous to that of Equation (406), and display the results in a manner similar to Comment upon your findings.