Recall the example from Exercise S8 of Chapter 5, where South Korea and Japan compete in the market for production of VLCCs. As in parts (a) and (b) of that exercise, the cost of building ships is $30 (million) in each country, and the demand for ships is
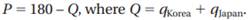
(a) Previously, we found the Nash equilibrium for the game. Now find the collusive outcome. What total quantity should be set by the two countries in order to maximize their joint profit?
(b) Suppose the two countries produce equal quantities of VLCCs, so that they earn equal shares of this collusive profit. How much profit would each country earn? Compare this profit with the amount they would earn in the Nash equilibrium.
(c) Now suppose the two countries are in a repeated relationship. Once per year, they choose production quantities, and each can observe the amount its rival produced in the previous year. They wish to cooperate to sustain the collusive profit levels found in part (b). In any one year, one of them can defect from the agreement. If one of them holds the quantity at the agreed level, what is the best defecting quantity for the other? What are the resulting profits?
(d) Write down a matrix that represents this game as a prisoners’ dilemma.
(e) For what interest rates will collusion be sustainable when the two countries use grim (defect forever) strategies?