Please help me solve this question as soon as possible (Applied Mathematics in Chemical Engineering)
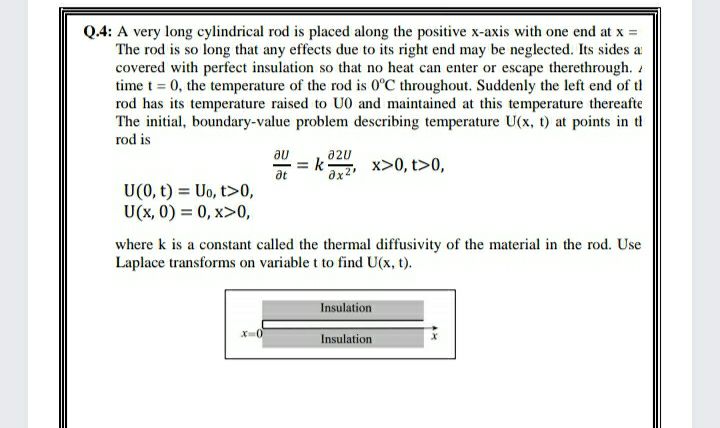
0, t>0, at U(0, t) = Uo, t>0, U(x, 0) = 0, x>0, where k is a constant called the thermal diffusivity of the material in the rod. Use Laplace transforms on variable t to find U(x, t). Insulation X-0 Insulation "/>
Extracted text: Q.4: A very long cylindrical rod is placed along the positive x-axis with one end at x = The rod is so long that any effects due to its right end may be neglected. Its sides a covered with perfect insulation so that no heat can enter or escape therethrough. time t = 0, the temperature of the rod is 0°C throughout. Suddenly the left end of tl rod has its temperature raised to U0 and maintained at this temperature thereafte The initial, boundary-value problem describing temperature U(x, t) at points in ti rod is a2u au = k dx2" x>0, t>0, at U(0, t) = Uo, t>0, U(x, 0) = 0, x>0, where k is a constant called the thermal diffusivity of the material in the rod. Use Laplace transforms on variable t to find U(x, t). Insulation X-0 Insulation