Prove that the properties of determinants are true by solving the following: -1 1 3 1 -5 -5 -1 -4 4 -4 4 3 -7 -4 4 3 A = 2 -5 B1 B2 -3 -6 -6 3 -6 -6 5 6 -3 -2] 6- 4 -4 -3 1 4 -4 -3. -2 -3 -2 -4 -6 k =...

PLEASE ANSWER
LETTER C

PLEASE SHOW COMPLETE DETAILED SOLUTION AND WRITE LEGIBLY. PLEASE NO SHORTCUTS I NEED COMPLETE.

Extracted text: Prove that the properties of determinants are true by solving the following: -1 1 3 1 -5 -5 -1 -4 4 -4 4 3 -7 -4 4 3 A = 2 -5 B1 B2 -3 -6 -6 3 -6 -6 5 6 -3 -2] 6- 4 -4 -3 1 4 -4 -3. -2 -3 -2 -4 -6 k = -2 C = 1 7 -1 D : 1 2 3 4 -1 3 6. -21] a. Using Matrix [A] prove that the Property 1 and 2 of determinant are true. b. Using Matrix [C] and 'k' prove that Property 3 of determinant is true. c. Using Matrix [D] prove that Property 4 of determinant is true. d. Using Matrix [B1] and [B2] prove that by solving the determinant of these matrices separately and getting its sum will give the same answer with the use of Property 5. NOTE: Use your convenient method when solving the determinant of the given matrices.

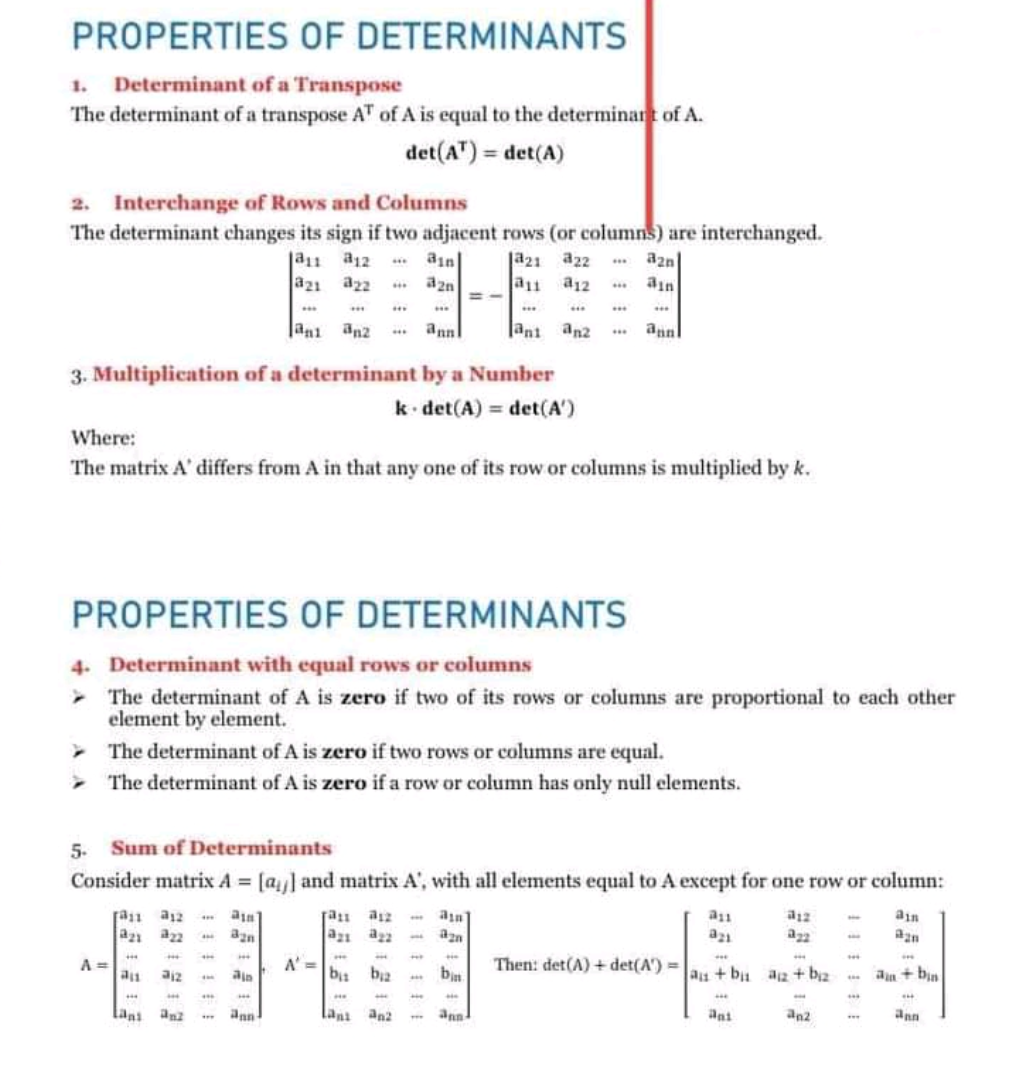
The determinant of A is zero if two rows or columns are equal. The determinant of A is zero if a row or column has only null elements. 5. Sum of Determinants Consider matrix A = [a and matrix A', with all elements equal to A except for one row or column: ran a12 an] a2n a2 an ain azn A = A' = b bz Then: det(A) + det(A') = an + bu az + bi2 an + bin ... Lani ann lan an2 ann an2 ann "/>
Extracted text: PROPERTIES OF DETERMINANTS 1. Determinant of a Transpose The determinant of a transpose AT of A is equal to the determinant of A. det(A") = det(A) 2. Interchange of Rows and Columns The determinant changes its sign if two adjacent rows (or columns) are interchanged. ja1 a12 a21 az2 ** ain ** azn ja21 a22 a1 a12 aznl ..* annl |ani an2 *** an 3. Multiplication of a determinant by a Number k det(A) = det(A') Where: The matrix A' differs from A in that any one of its row or columns is multiplied by k. PROPERTIES OF DETERMINANTS 4. Determinant with equal rows or columns - The determinant of A is zero if two of its rows or columns are proportional to each other element by element. > The determinant of A is zero if two rows or columns are equal. The determinant of A is zero if a row or column has only null elements. 5. Sum of Determinants Consider matrix A = [a and matrix A', with all elements equal to A except for one row or column: ran a12 an] a2n a2 an ain azn A = A' = b bz Then: det(A) + det(A') = an + bu az + bi2 an + bin ... Lani ann lan an2 ann an2 ann