What is the minimum number of passengers to contract the virus?
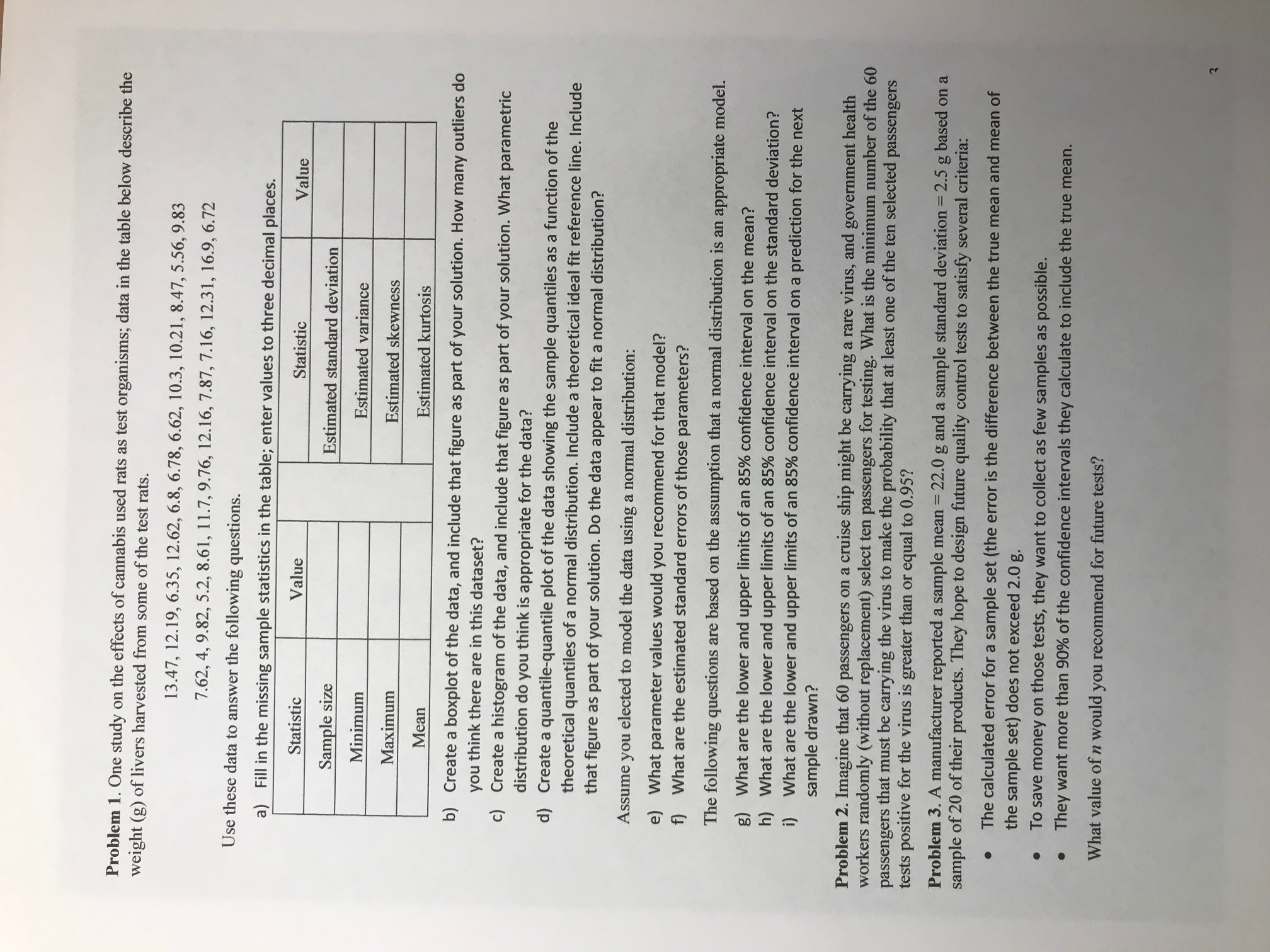
Extracted text: Problem 1. One study on the effects of cannabis used rats as test organisms; data in the table below describe the weight (g) of livers harvested from some of the test rats. 13.47, 12.19, 6.35, 12.62, 6.8, 6.78, 6.62, 10.3, 10.21, 8.47, 5.56, 9.83 7.62, 4, 9.82, 5.2, 8.61, 11.7, 9.76, 12.16, 7.87, 7.16, 12.31, 16.9, 6.72 Use these data to answer the following questions. a) Fill in the missing sample statistics in the table; enter values to three decimal places. Statistic Value Statistic Value Sample size Estimated standard deviation Minimum Estimated variance Maximum Estimated skewness Mean Estimated kurtosis b) Create a boxplot of the data, and include that figure as part of your solution. How many outliers do you think there are in this dataset? c) Create a histogram of the data, and include that figure as part of your solution. What parametric distribution do you think is appropriate for the data? d) Create a quantile-quantile plot of the data showing the sample quantiles as a function of the theoretical quantiles of a normal distribution. Include a theoretical ideal fit reference line. Include that figure as part of your solution. Do the data appear to fit a normal distribution? Assume you elected to model the data using a normal distribution: e) What parameter values would you recommend for that model? f) What are the estimated standard errors of those parameters? The following questions are based on the assumption that a normal distribution is an appropriate model. g) What are the lower and upper limits of an 85% confidence interval on the mean? h) What are the lower and upper limits of an 85% confidence interval on the standard deviation? i) What are the lower and upper limits of an 85% confidence interval on a prediction for the next sample drawn? Problem 2. Imagine that 60 passengers on a cruise ship might be carrying a rare virus, and government health workers randomly (without replacement) select ten passengers for testing. What is the minimum number of the 60 passengers that must be carrying the virus to make the probability that at least one of the ten selected passengers tests positive for the virus is greater than or equal to 0.95? Problem 3. A manufacturer reported a sample mean = sample of 20 of their products. They hope to design future quality control tests to satisfy several criteria: 22.0 g and a sample standard deviation = 2.5 g based on a The calculated error for a sample set (the error is the difference between the true mean and mean of the sample set) does not exceed 2.0 g. To save money on those tests, they want to collect as few samples as possible. They want more than 90% of the confidence intervals they calculate to include the true mean. What value of n would you recommend for future tests?