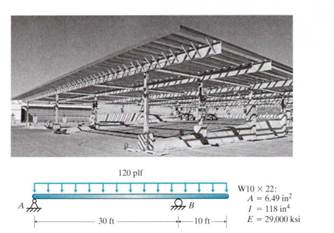
Our firm has been hired to design a sun screen to protect cars on the top level of a parking garage. The design team has determined the ideal structural system: two beams will extend from a central column, pass over exterior columns, and cantilever over the parking spots. For aesthetic reasons, the architect wants us to limit the dead load deflection of the beam to no more than 1 inch. Determine the peak vertical deflection for one of the beams given our current choice of beam section. The distributed load shown already includes the self-weight of the beam.
a. Guess the anticipated deflected shape.
b. Estimate the peak deflection by ignoring the distributed load on the cantilevered end. Will this provide an upper bound, a lower bound, or just an approximation for the actual peak vertical displacement? Justify your answer.
c. Derive the expressions for the slope and displacement due to dead load along the entire beam. Determine the peak displacement and its location (creating a table of values is acceptable for this).
d. Plot the expressions for the slope and displacement.
e. Identify at least four features of the expressions for the slope and displacement that suggest you have a reasonable answer.
f. Evaluate the displacement prediction using your results from parts (b) and (e).
g. Comment on why your guess in part (a) was or was not close to the solution in part (d).