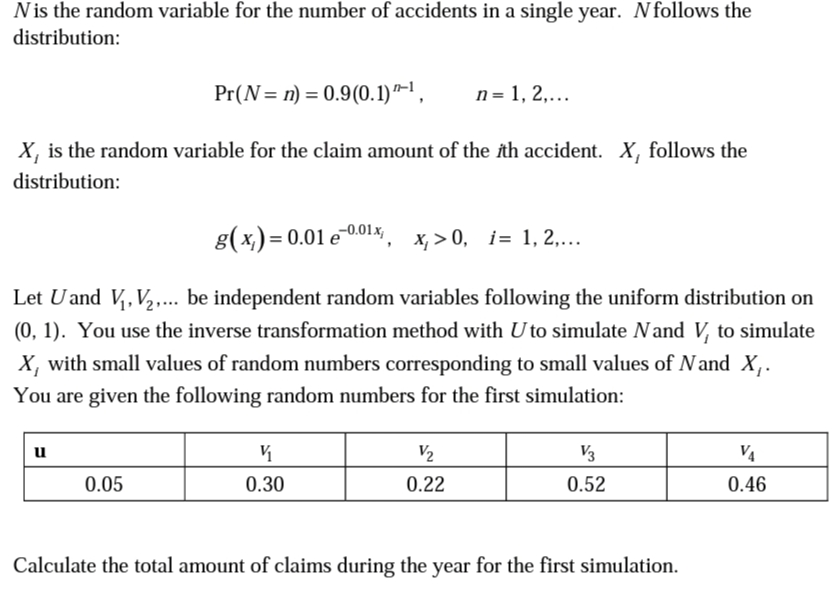
0, i= 1, 2,... Let Uand V,, V,... be independent random variables following the uniform distribution on (0, 1). You use the inverse transformation method with U to simulate Nand V, to simulate X, with small values of random numbers corresponding to small values of Nand X,. You are given the following random numbers for the first simulation: u V2 V3 V4 0.05 0.30 0.22 0.52 0.46 Calculate the total amount of claims during the year for the first simulation. "/>
Extracted text: Nis the random variable for the number of accidents in a single year. Nfollows the distribution: Pr(N= n) = 0.9(0.1)"-' n = 1, 2,... X, is the random variable for the claim amount of the ith accident. X, follows the distribution: g(x,) = 0.01 e 0.01x, x,>0, i= 1, 2,... Let Uand V,, V,... be independent random variables following the uniform distribution on (0, 1). You use the inverse transformation method with U to simulate Nand V, to simulate X, with small values of random numbers corresponding to small values of Nand X,. You are given the following random numbers for the first simulation: u V2 V3 V4 0.05 0.30 0.22 0.52 0.46 Calculate the total amount of claims during the year for the first simulation.