Need help with this question. Thank you :)
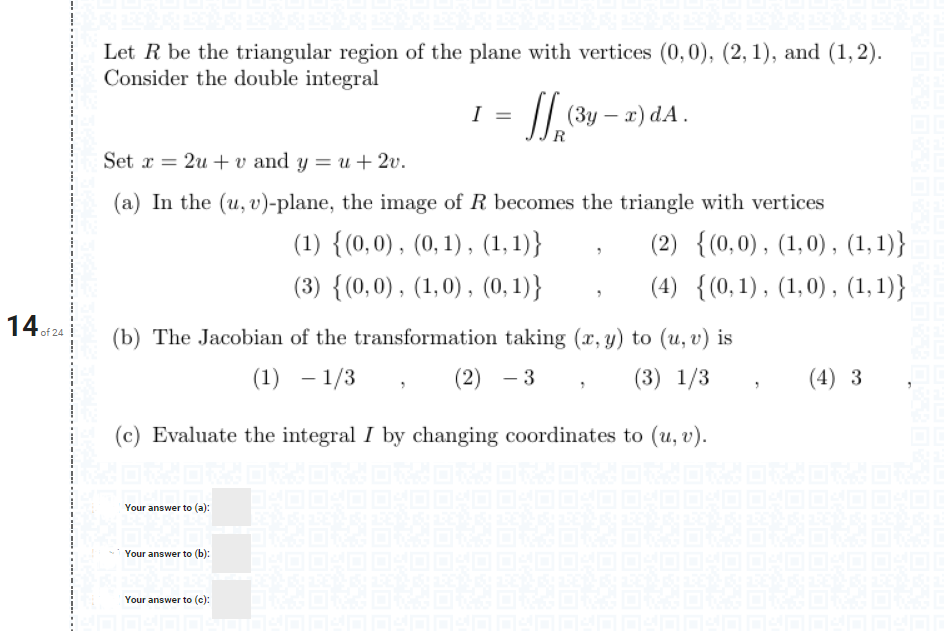
Extracted text: Let R be the triangular region of the plane with vertices (0, 0), (2, 1), and (1, 2). Consider the double integral I = I| (3y – a) dA. Set x = 2u + v and y = u+ 2v. (a) In the (u, v)-plane, the image of R becomes the triangle with vertices (1) {(0,0), (0, 1) , (1,1)} (2) {(0,0), (1,0) , (1,1)} (3) {(0,0), (1,0) , (0,1)} (4) {(0,1), (1,0) , (1, 1)} 14of24 (b) The Jacobian of the transformation taking (x, y) to (u, v) is (1) – 1/3 (2) - 3 (3) 1/3 (4) 3 (c) Evaluate the integral I by changing coordinates to (u, v). Your answer to (a): Your answer to (b): Your answer to (c):