Show me the steps of determine red and it complete
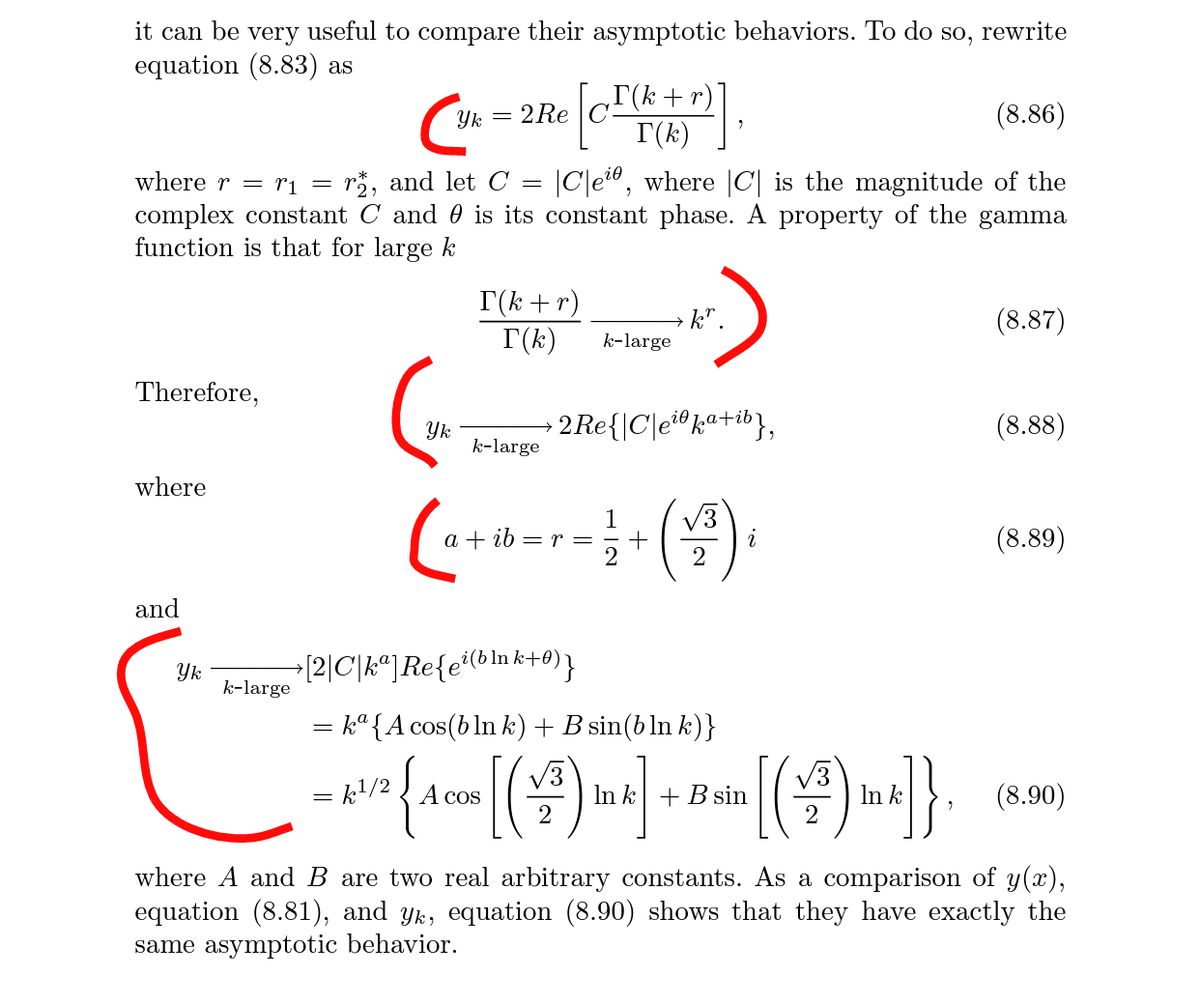
Extracted text: it can be very useful to compare their asymptotic behaviors. To do so, rewrite equation (8.83) as T(k+r) I(k) Yk 2 Re (8.86) where r = ri = r*, and let C = |C|e", where |C| is the magnitude of the complex constant C and 0 is its constant phase. A property of the gamma function is that for large k T(k +r) T(k) k". k-large (8.87) Therefore, Yk k-large • 2Re{\C\e®k«+ib}, (8.88) where 3 1 ib = r = -+ 2 (8.89) 2 and Yk k-large +[2|C\k"]Re{e'(b\nk+0)} k° {A cos(b ln k) +B sin(b ln k)} [(4). kl/2 A cos In k + B sin In k (8.90) where A and B are two real arbitrary constants. As a comparison of y(x), equation (8.81), and yk, equation (8.90) shows that they have exactly the same asymptotic behavior.
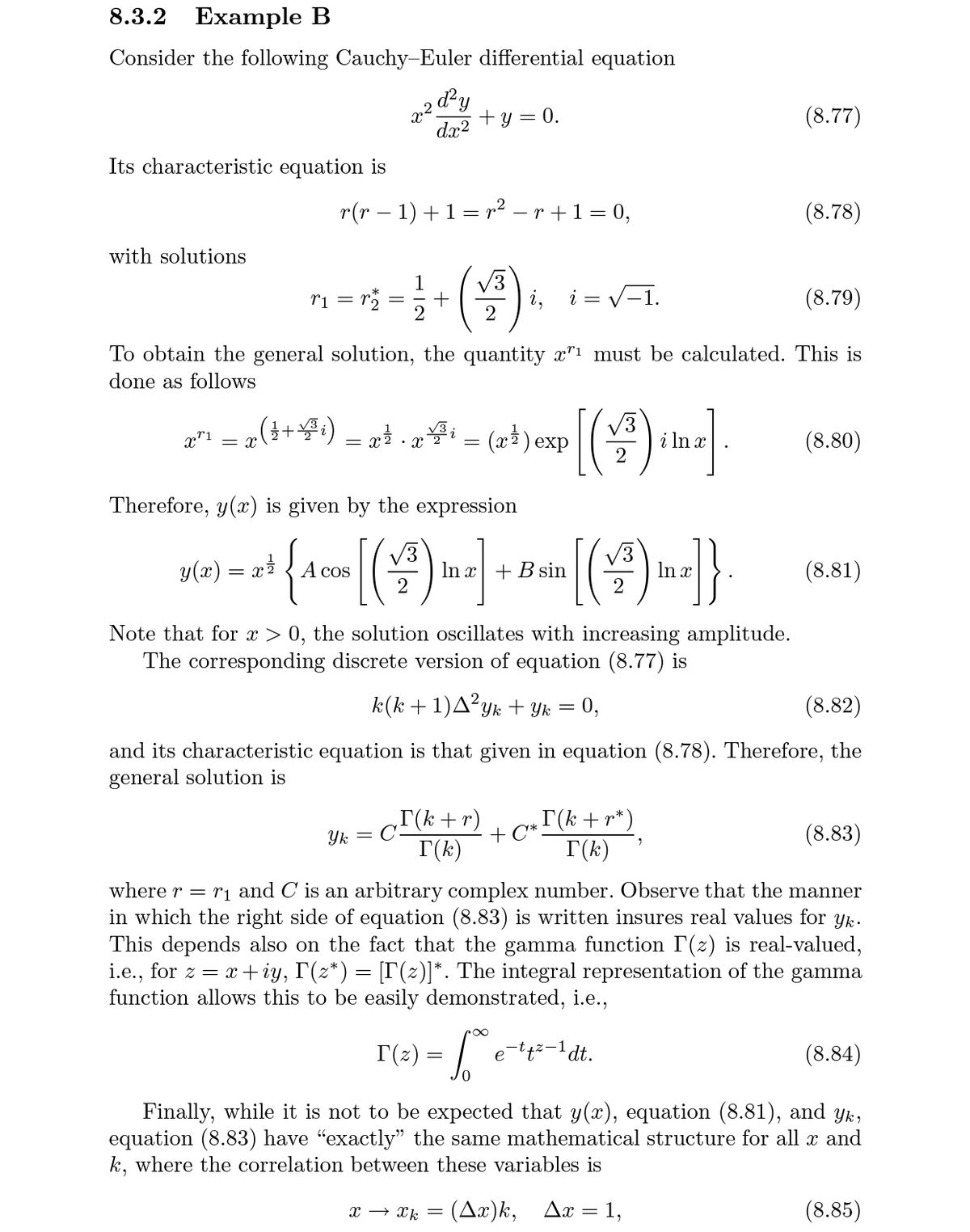
0, the solution oscillates with increasing amplitude. The corresponding discrete version of equation (8.77) is k(k + 1)A²yk + Yk 0, (8.82) and its characteristic equation is that given in equation (8.78). Therefore, the general solution is ,r(k+r) I(k) T(k +r*) + C* T(k) Yk = (8.83) where r = r1 and C is an arbitrary complex number. Observe that the manner in which the right side of equation (8.83) is written insures real values for yk. This depends also on the fact that the gamma function T(z) is real-valued, i.e., for z = x+ iy, I'(z*) = [T'(2)]*. The integral representation of the gamma function allows this to be easily demonstrated, i.e., I(2) = | e-t-1dt. (8.84) Jo Finally, while it is not to be expected that y(x), equation (8.81), and yk, equation (8.83) have "exactly" the same mathematical structure for all x and k, where the correlation between these variables is x → Xk = (Ax)k, Ax = 1, (8.85) %3D "/>
Extracted text: 8.3.2 Example B Consider the following Cauchy-Euler differential equation d²y + y = 0. dx2 (8.77) Its characteristic equation is r(r – 1) +1= r2 – r +1= 0, (8.78) - with solutions 1 = ra i, = V-1. (8.79) ri = + 2 To obtain the general solution, the quantity x"1 must be calculated. This is done as follows (a) exp V3 x"1 In x (8.80) = x = X • x Therefore, y(x) is given by the expression y(x) = x A cos In x + B sin In x (8.81) Note that for x > 0, the solution oscillates with increasing amplitude. The corresponding discrete version of equation (8.77) is k(k + 1)A²yk + Yk 0, (8.82) and its characteristic equation is that given in equation (8.78). Therefore, the general solution is ,r(k+r) I(k) T(k +r*) + C* T(k) Yk = (8.83) where r = r1 and C is an arbitrary complex number. Observe that the manner in which the right side of equation (8.83) is written insures real values for yk. This depends also on the fact that the gamma function T(z) is real-valued, i.e., for z = x+ iy, I'(z*) = [T'(2)]*. The integral representation of the gamma function allows this to be easily demonstrated, i.e., I(2) = | e-t-1dt. (8.84) Jo Finally, while it is not to be expected that y(x), equation (8.81), and yk, equation (8.83) have "exactly" the same mathematical structure for all x and k, where the correlation between these variables is x → Xk = (Ax)k, Ax = 1, (8.85) %3D