(b) Compressor (for gases): Tds = dh – vdP, ds = 0 (isentropic) || From Tds relations vdP = dh -[ vdP -S dh=-(h, – h,) W, = - rev State 1 (Table A-5): P =100 kPa, Sat. vapour s, =7.3589 kJ/kgK S1 h =...

For the given question and solution, for part (b), finding the work input  of the saturated vapour at the inlet state,

why couldnt i  use the same formula as part (a) Win = v*(change in pressure) where the v would be the saturated vapour specific volume instead of saturated fluid volume??

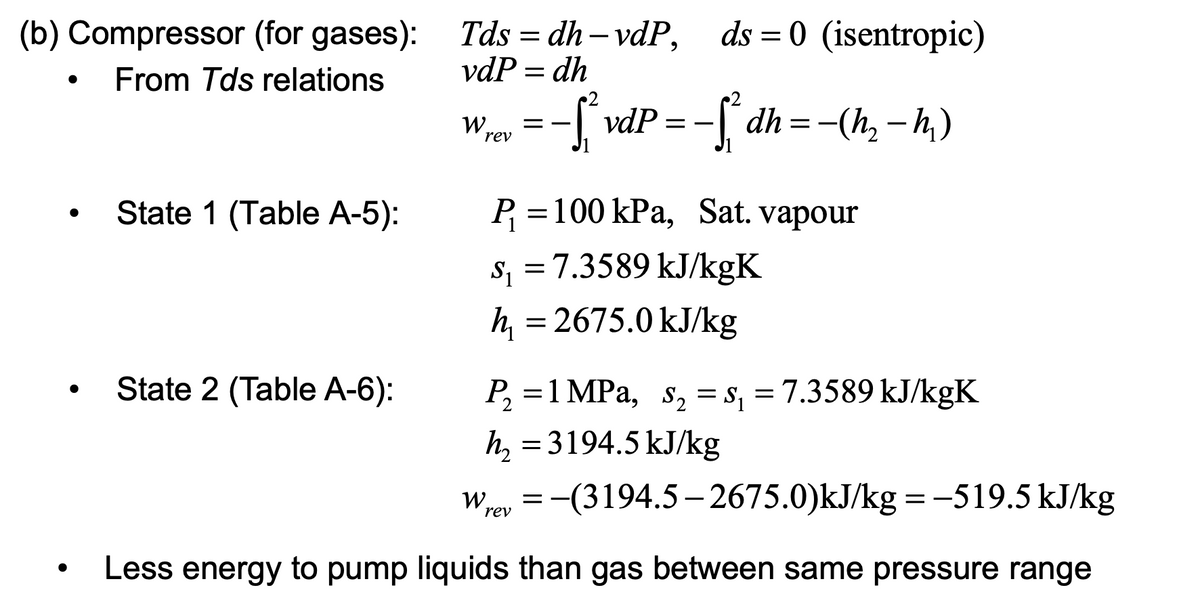
Extracted text: (b) Compressor (for gases): Tds = dh – vdP, ds = 0 (isentropic) || From Tds relations vdP = dh -[ vdP -S dh=-(h, – h,) W, = - rev State 1 (Table A-5): P =100 kPa, Sat. vapour s, =7.3589 kJ/kgK S1 h = 2675.0 kJ/kg State 2 (Table A-6): P, =1 MPa, s, = s, = 7.3589 kJ/kgK h, = 3194.5 kJ/kg Wroy = -(3194.5- 2675.0)kJ/kg = -519.5 kJ/kg rev Less energy to pump liquids than gas between same pressure range


Extracted text: Determine the pump (compressor) work input required to compress steam isentropically from 100 kPa to 1 MPa, assuming that the steam exists as (a) saturated liquid and (b) saturated vapour at the inlet state. T P2 = 1 MPa Process is isentropic: P, = 1 MPa 1 MPa (a) Pump (for liquid, v = const.): PUMP COMPRESSOR (b) V1 = Vf@100kPa = 0.001043 m'/kg (a) 100 kPa P = 100 kPa P = 100 kPa W,ey = -[ vdP = -v, (P, -R) %3| (b) Compressing (a) Compressing a liquid rev a vapor 1 kJ =-(0.001043 m/kg)[(1000-100) kPa] =-0.94 kJ/kg W, 3 1kPa · m rev