According to the Colebrook equation, the friction factor
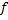
for turbulent flow in a pipe is found by solving
where
and
are constants that satisfy 0
<><>
= 1/
, this equation can be rewritten as
where 0
.
(a) Sketch the two functions in (2.31) for 0
. Use this to explain why there is only one solution, and that it is in the interval 0
<>10(1/).
In the rest of the problem assume that
= 10−2
and
= 10−4, which are typical values for these constants.
(b) What does (2.10) reduce to for (2.31)? Based on part (a), what would be a good choice for
0? Make sure to explain why.
(c) Based on part (a), what would be a good choice for
0
and
0
when using the bisection method to solve (2.31)? Make sure to explain why.
(d) Based on part (a), what would be a good choice for
0
and
1
when using the secant method to solve (2.31)? Make sure to explain why.
(e) Use one of the methods from (b)–(d) to solve (2.31), and from this determine the value of
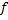. Make sure to state which method was used, why you made this choice, and what error condition you used to stop the calculation.