Are my answers correct? Please notice the repeated alphabetical choices that are available as answers because they can be confusing. For part 3, I'm not sure if its correct because there are 2 letters of D, but they both seem correct to me. Do correct me if I'm wrong
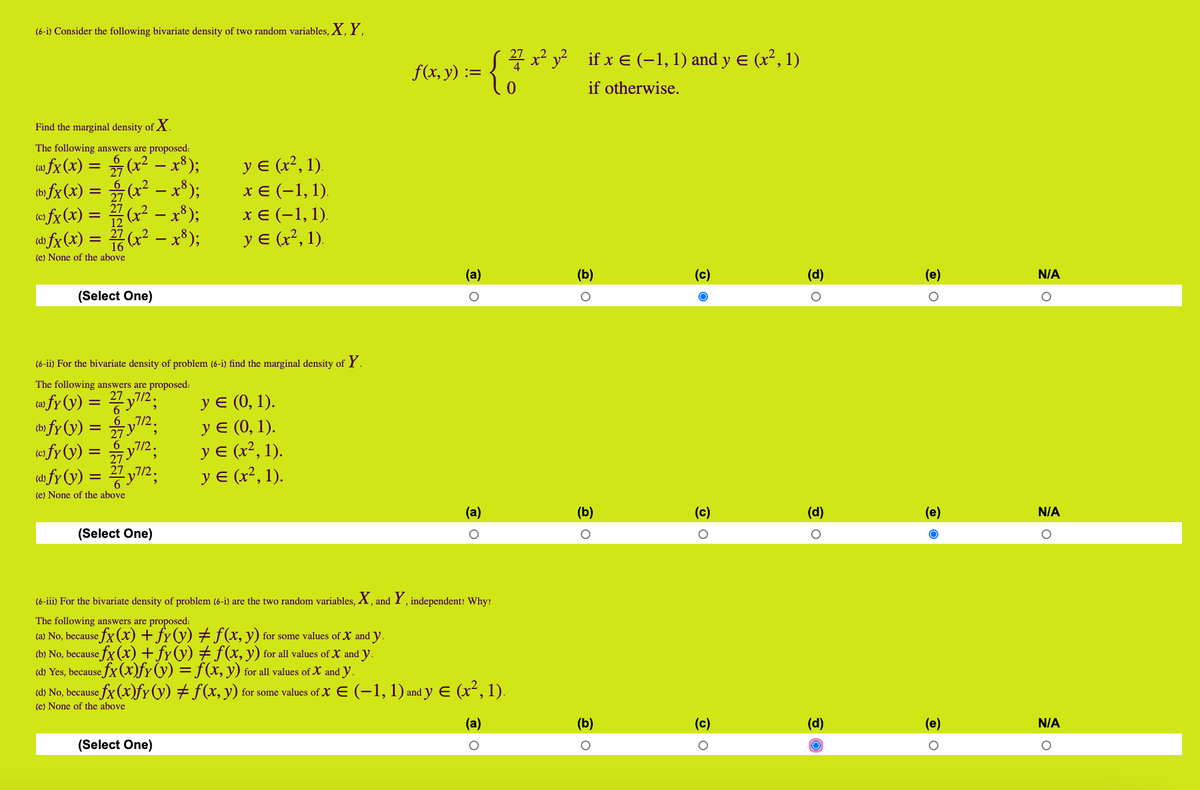
Extracted text: (6-i) Consider the following bivariate density of two random variables, X, Y. S7 x? y? if x e (–1, 1) and y E (x², 1) f(x, y) : if otherwise. Find the marginal density of X. The following answers are proposed: y E (x², 1). ca) fx (x) = (x2 - x*); (» fx(x) = (x² – x³); (© fx (x) = (x² - x³); dh fx (x) = 7(x² – x³); 6. 27 хе (-1,1). - хе (-1,1). y E (x², 1). 12 16 (e) None of the above (а) (b) (c) (d) (e) N/A (Select One) (6-ii) For the bivariate density of problem (6-i) find the marginal density of Y. The following answers are proposed: Уе (0, 1). У (0, 1). У (?, 1). yε α?, 1). ca fy (y) = " y72; tb fy (y) = y2; 6 ,7/2 (© fy(y) = y2; ca) fy (y) = 7 y2; (e) None of the above (а) (b) (c) (d) (e) N/A (Select One) (6-iii) For the bivariate density of problem (6-i) are the two random variables, X, and Y, independent: Why: The following answers are proposed: (a) No, because efx(x) + fy(y) #f(x, y) for some values of X and y. (b) No, because fx (X) + fy(y) # f(x, y) for all values of X and y. (d) Yes, because fx (Xx)fy(y) = f(x, y) for all values of X and y. (d) No, because fx (x)fr(y) # f(x, y) for some values of X E (-1, 1) and y E (x², 1). (e) None of the above (a) (b) (c) (d) N/A (Select One)