6. By referring to Example 46.11, actually express the gcd 23 in the form 2(22,471) + µ(3,266) for 2, µ e Z. [Hint: From the next to the last line of the computation in Example 46.11, 23 = (138)3 –...

Section 46 number 8. Use number 6 and 7 to help and 46.11

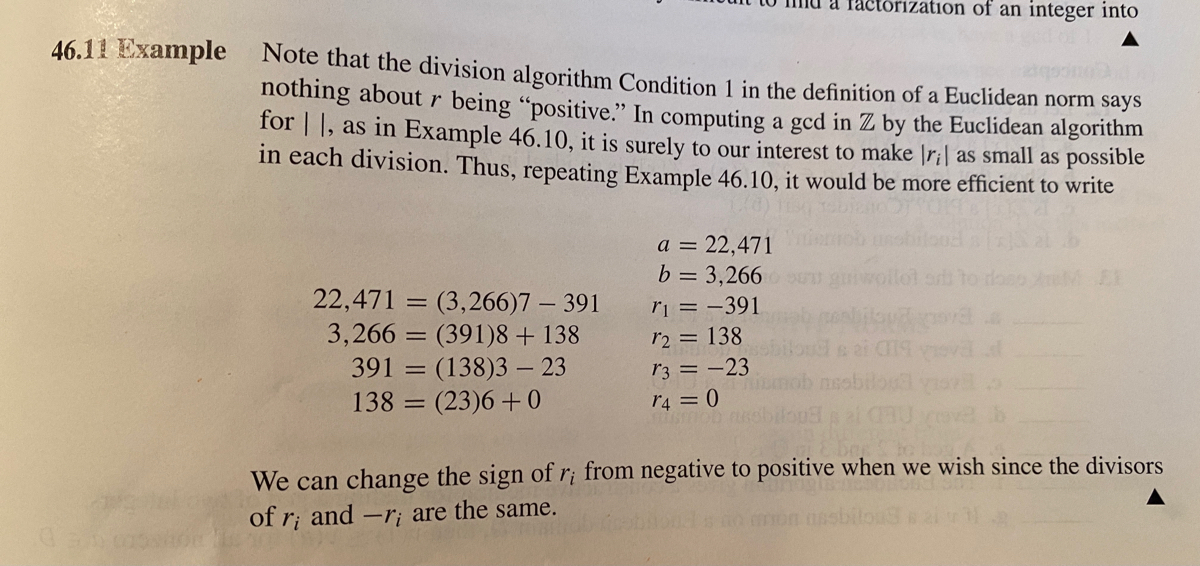
Extracted text: 6. By referring to Example 46.11, actually express the gcd 23 in the form 2(22,471) + µ(3,266) for 2, µ e Z. [Hint: From the next to the last line of the computation in Example 46.11, 23 = (138)3 – 391. From the line before that, 138 = 3,266 – (391)8, so substituting, you get 23 = [3,266 – (391)8]3 – 391, and so on. That is, work your way back up to actually find values for and u.] 7. Find a gcd of 49,349 and 15,555 in Z. 8. Following the idea of Exercise 6 and referring to Exercise 7, express the positive gcd of 49,349 and 15,555 in Z in the form 2(49,349) + µ(15,555) for 2, µ e Z.


Extracted text: factórization of an integer into 46.11 Example Note that the division algorithm Condition 1 in the definition of a Euclidean norm says nothing about r being "positive." In computing a gcd in Z by the Euclidean algorithm for | |, as in Example 46.10, it is surely to our interest to make |r¡| as small as possible in each division. Thus, repeating Example 46.10, it would be more efficient to write a = 22,471 b = 3,266 a to 22,471 = (3,266)7 – 391 3,266 = (391)8 + 138 391 = (138)3 – 23 138 = (23)6+0 ri = -391 r2 = 138 ai CS eva r3 = -23 r4 =0 We can change the sign of r¡ from negative to positive when we wish since the divisors of r; and -r; are the same.