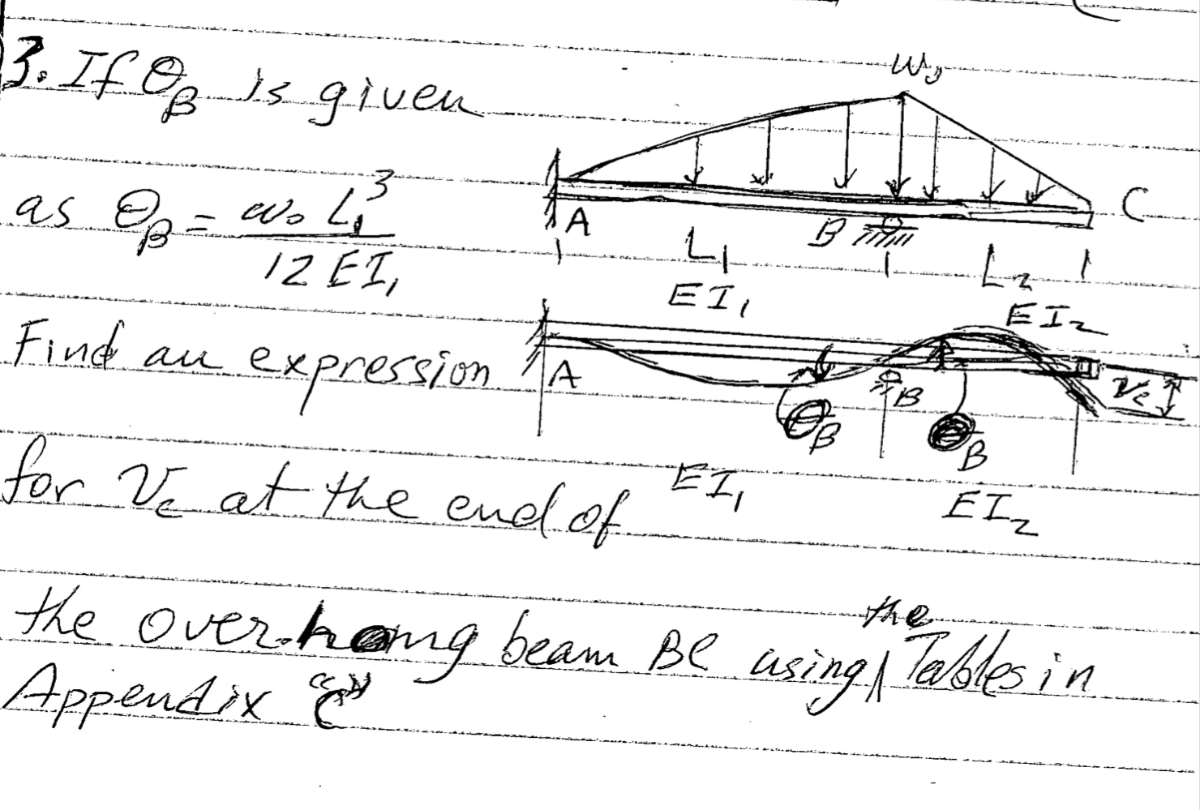
Extracted text: 3.IfOis glven Wo Li 12 EI, A as Eg= EII EIz expression TA for Ve at the enelof. Find au --the the overhomg beam Be using Tables in Appendix gd
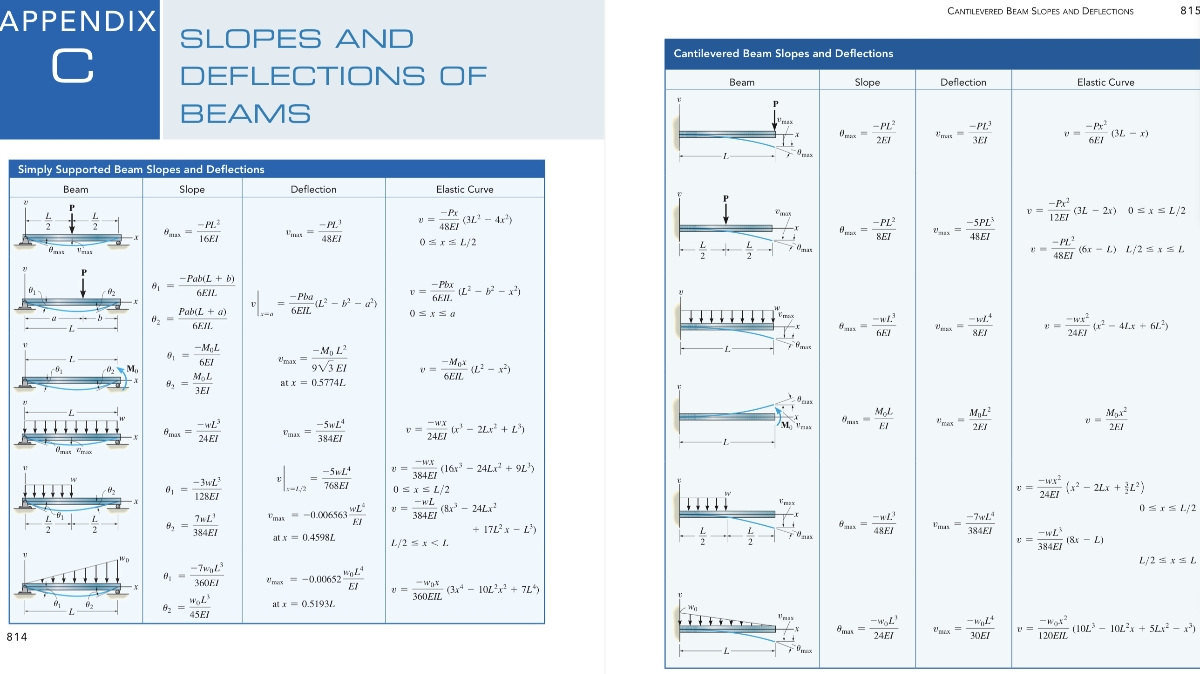
Extracted text: APPENDIX CANTILEVERED BEAM SLOPES AND DEFLECTIONS 815 SLOPES AND Cantilevered Beam Slopes and Deflections DEFLECTIONS O F Beam Slope Deflection Elastic Curve BEAMS "max -PL -PL' Oman (3L - x) 6EI 2EI 3EI Simply Supported Beam Slopes and Deflections Beam Slope Deflection Elastic Curve -P 12EL 3L - 2x) 0sxS L/2 P -Px (31 - 4x) 48EI -5PL -PL. -PL. -PL? e = SEI 16EI 48EI 48EI 4RET (6x - L) L/2 sxSI, 48EI -Pab(L + b) - Pbx 6EIL L - - GEIL. -a 12 - - l' - - a') 6EIL Pab(L + a) -wl.' (x2 - 41x + 62) 24EI 6EIL. SEJ -ML -M, L 6EI "max = -Mox 9V3 EI (L? - x) 6EIL 8, = 3EI at x = 0.5774L Mol -wL -5wL -WX r' - 2Lx + L') M Vnas El 2EI Bmas Pmax 24EI 24EI 384EI -WN (16r - 24Lx + 9L') -SwL 384EI - 3wl 768EI 0, = 0SxS L/2 24EI - 2Lx + L) 128EI -wL (8x 384EI wL 2 = 24LX 7wL' Fman - -0.006563 EI -7wl" 2 8, = + 17Lx - L') 48EI Emas 384EI 384EI at x = 0.4598L L/2 sx