(18) Find the ratio in which the join of the points A(12, –5) and B(3, 4) is cut by the line r (3i – 11j) = 0. Show that the line joining the origin to the point dividing AB externally in the same...

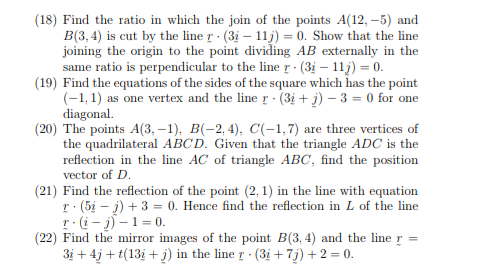
Extracted text: (18) Find the ratio in which the join of the points A(12, –5) and B(3, 4) is cut by the line r (3i – 11j) = 0. Show that the line joining the origin to the point dividing AB externally in the same ratio is perpendicular to the line r - (3i – 11j) = 0. (19) Find the equations of the sides of the square which has the point (-1, 1) as one vertex and the line r (3i + j) – 3 = 0 for one diagonal. (20) The points A(3, –1), B(-2, 4), C(-1,7) are three vertices of the quadrilateral ABCD. Given that the triangle ADC is the reflection in the line AC of triangle ABC, find the position vector of D. (21) Find the reflection of the point (2, 1) in the line with equation r: (5i – j) + 3 = 0. Hence find the reflection in L of the line r:(i - j) –1 = 0. (22) Find the mirror images of the point B(3, 4) and the line 3i + 4j + t(13i + j) in the line r (3i +7j) +2 = 0.


Extracted text: (1) Find the perpendicular distances of (a) the point (-6, 2) from the line r (5i+ 12j) = 7; (b) the point (3, 4) from the line r - (3i + 4j) = 10; (e) the point (2, -3) from the line r (i – 5j) – 1 = 0; (d) the origin from the line r - (7ị – 3j) – 29 = 0. (2) Find the position vector of of the foot of the perpendicular from the point with position vector đị – 2j to the line r-(2i – 3) = 1. (3) Find the length of the altitude of the triangle A(2, 1), B(–1,3), C(-4, –3) from B to AC. (4) If Q is the foot of the perpendicular from (8,7) to the line joining A(3, 6) to B(9, 2), find the position vector of Q, and the ratio in which Q divides AB. (5) P and Q are the feet of the perpendiculars from A(0, 5) and B(-6,0) to the line r:(-i+2;) = 4. Find (a) the equations of AP and BQ. the distance PQ, (c) the position vector of the point of intersection of AQ with the r-axis. (6) Find the distances between the following pairs of parallel lines: (a) r - (i + j) + 7 = 0, r · (i +¿) – 11 = 0; (b) r- (2i – 3) + 6 = 0, r · (di – 6) +5 = 0; (c) r • (4i – 3) – 9 = 0, r · (di – 3) – 24 = 0; (d) r = i+j+s(4i –i). r = 4ị + 5j +t(8į – 25) (7) Find the equations of the bisectors of the angles between the following pairs of lines and, in each case, discriminate between them: (a) r- (2i + j) + 4 = 0, r · (2i – 4j) – 7 = 0; (b) r- (3i + 4j) + 5 = 0, r - (5i + 12j) + 13 = 0; (d) r- (3i + 4) – 12 = 0, r - (3i – j) – 5 = 0; (e) r- (24i +7j) = 20, r- (di – 3) = 2. (8) Find the equation of the bisectors of the angles between the line joining the points with position vectors -3i – 9j. 2i + 3j and the line meeting the r- and y- axes at the points with position vectors 3i and 4j. 1 2 (9) Find the equation of the line, which is such that the axis of 1 bisects the angle between it and the line r · (2i + 5j) = 18. (10) If y = mx bisects an angle between the lines y = m,1, y = m3a, show that (m – m,)(1+ mm,) + (m – m,)(1+ mm,) = 0, and deduce that the two bisectors are given by the roots of the equation (m, + ma)m² + 2(1 – m,mą]m – (m, + m2) = 0. Show that the two values of m given by the above equation determine perpendicular lines. Show also that if m, = 1/m, then the bisectors are y =1, y = -1. (11) The position vectors of the vertices of a triangle are a = -i+j. b = Ti – 2j. c = 5i + 3j. Find the length of one side of the triangle and the length of the altitude to that side and hence compute the area of the triangle.