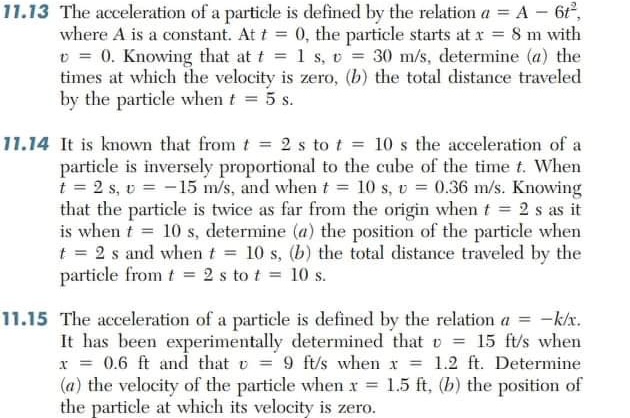
Extracted text: 11.13 The acceleration of a particle is defined by the relation a = A 6, where A is a constant. At t = 0, the particle starts at x = 8 m with E = 0. Knowing that at t = 1 s, v = 30 m/s, determine (a) the times at which the velocity is zero, (b) the total distance traveled by the particle when t = 5 s. 11.14 It is known that from t = 2 s to t = 10 s the acceleration of a particle is inversely proportional to the cube of the time t. When i = 2 s, v = -15 m/s, and when t = 10 s, v = 0.36 m/s. Knowing that the particle is twice as far from the origin when t = 2 s as it is when t 10 s, determine (a) the position of the particle when t = 2 s and when t = 10 s, (b) the total distance traveled by the particle from t = 2 s to t = 10 s. 11.15 The acceleration of a particle is defined by the relation a = -k/x. It has been experimentally determined that v = 15 ft/s when x = 0.6 ft and that v = 9 ft/s when x = 1.2 ft. Determine (a) the velocity of the particle when x = 1.5 ft, (b) the position of the particle at which its velocity is zero. %3D %3D