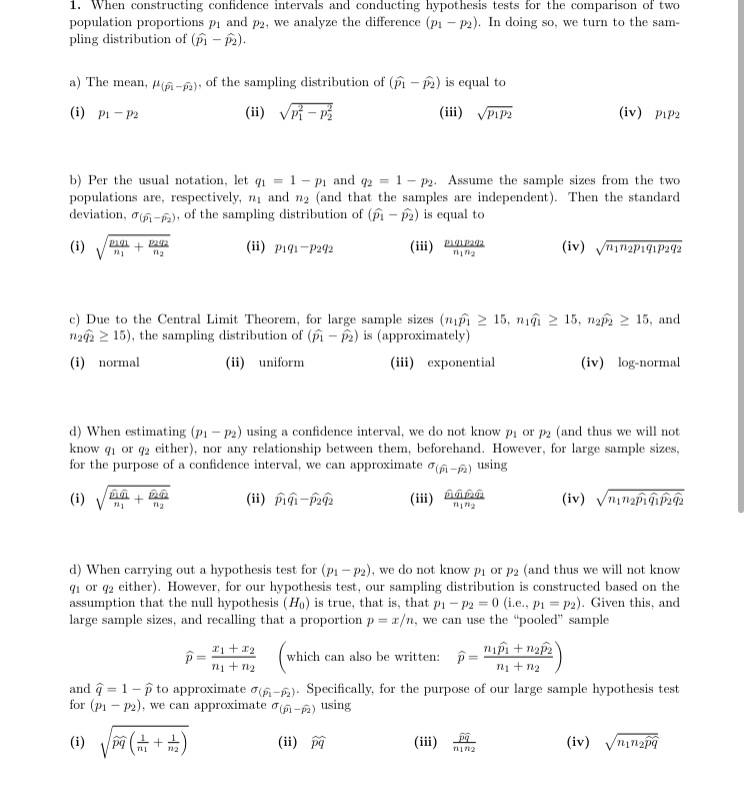
Extracted text: 1. When constructing confidence intervals and conducting hypothesis tests for the comparison of two population proportions pi and p2, we analyze the difference (p1 – P2). In doing so, we turn to the sam- pling distribution of (pi - P2). a) The mean, 4-A), of the sampling distribution of (i - P2) is equal to (i) Pi - P2 (ii) Vi - p (iii) VPIP2 (iv) PIP2 1- pi and q2 = 1- p2. Assume the sample sizes from the two b) Per the usual notation, let q1 = populations are, respectively, ni and n2 (and that the samples are independent). Then the standard deviation, o6-) of the sampling distribution of (fi - Pa) is equal to (i) (ii) p191-P242 (iii) DOPR (iv) yninap191P292 c) Due to the Central Limit Theorem, for large sample sizes (ni 2 15, niậi 2 15, na 2 15, and nz2 2 15), the sampling distribution of (- P) is (approximately) (i) normal (ii) uniform (iii) exponential (iv) log-normal d) When estimating (p1- P2) using a confidence interval, we do not know pi or p2 (and thus we will not know q1 or q2 either), nor any relationship between them, beforehand. However, for large sample sizes, for the purpose of a confidence interval, we can approximate oa-e) using (i) V (ii) piĝi-p2 (iii) d) When carrying out a hypothesis test for (p1 – P2), we do not know pi or p2 (and thus we will not know q1 or q2 either). However, for our hypothesis test, our sampling distribution is constructed based on the assumption that the null hypothesis (Ho) is true, that is, that p1- P2 = 0 (i.e., p1 = p2). Given this, and large sample sizes, and recalling that a proportion p = x/n, we can use the "pooled" sample which can also be written: p n1 + n2 ni + n2 and ĝ = 1- p to approximate o(6-). Specifically, for the purpose of our large sample hypothesis test for (pi - p2), we can approximate o6-) using () V( +) (ii) pa (ii) (iv) n2