Data were collected to explain the satisfaction rating a caller gives a customer representative based on the amount of time the caller is on hold (hold time is expressed in minutes) *COMPLETE THE TABLE BELOW*
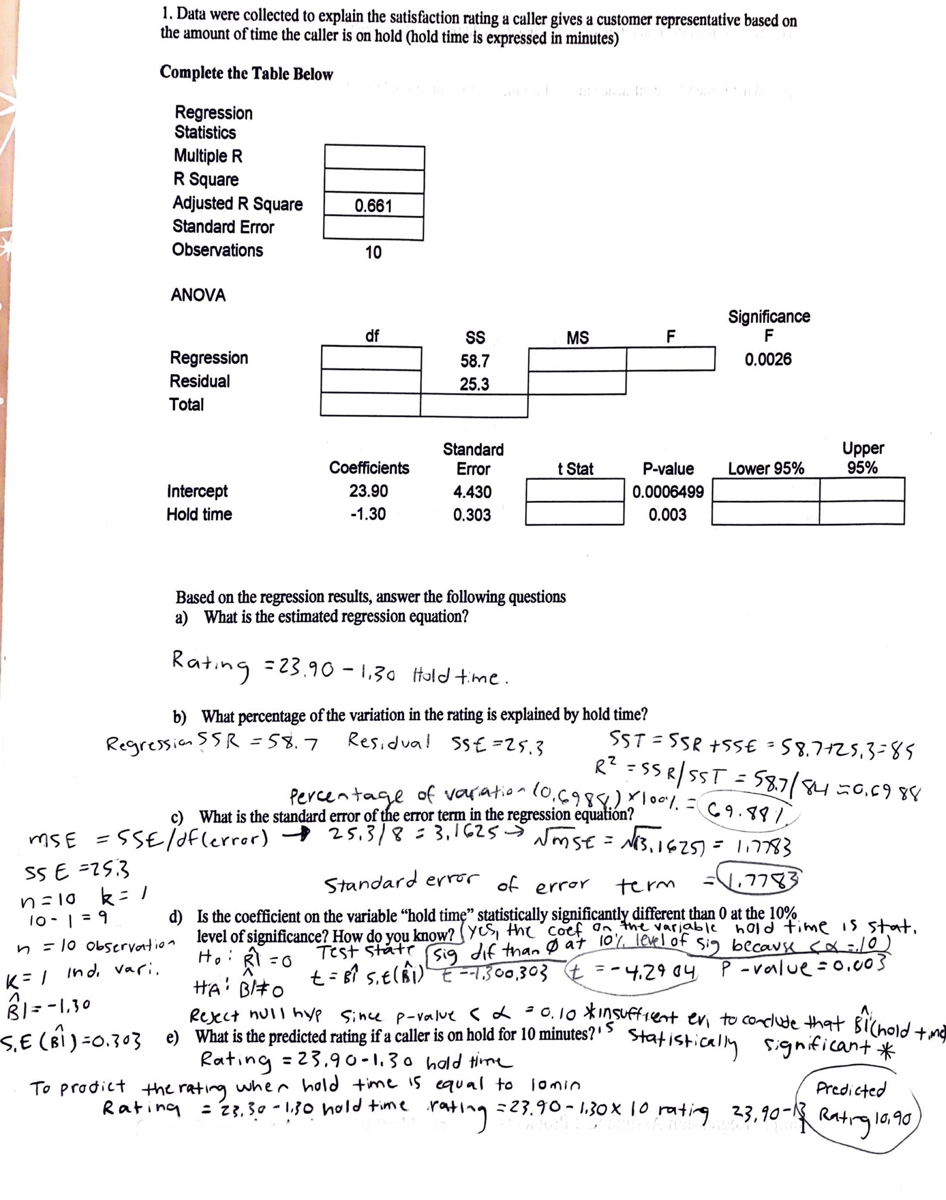
Extracted text: 1. Data were collected to explain the satisfaction rating a caller gives a customer representative based on the amount of time the caller is on hold (hold time is expressed in minutes) Complete the Table Below Regression Statistics Multiple R R Square Adjusted R Square 0.661 Standard Error Observations 10 ANOVA Significance F df MS Regression Residual 58.7 0.0026 25.3 Total Standard Error Upper 95% Coefficients t Stat P-value Lower 95% Intercept 23.90 4.430 0.0006499 Hold time -1.30 0.303 0.003 Based on the regression results, answer the following questions a) What is the estimated regression equation? Rating =23.90 - 1,30 told time. b) What percentage of the variation in the rating is explained by hold time? Residual Ss€ =25.3 SST = 5SR +55€ - 58.7725,3=85 R? = SSR/SST = 587/84. Regression sSR =58.7 Percentage of varation (o.c98)100. = c9.997 What is the standard error of the error term in the regression equatión? mSE =5SE/aflerror) $25.318=3,1625 sE = NB,1625) = 1,7783 SS E =25.3 Standard error of error 1,7783 term n=10 10-| = 9 d) Is the coefficient on the variable “hold timę" statistically significantly different than 0 at the 10% level of significance? How do you know? (yeS, the coef on tne varjable hold time is stat. Ho RI =0 HA: BI#O Rexct null hye since p-valueca0.10 *nsUffient ev to conclude that Bichold +na n = 10 observation Test statr K=I Ind. vari. B1= -1.30 SE(BI)=0.303 e) What is the predicted rating if a caller is on hold for 10 minutes?' ? Statisticaly significant * -300,303 t -4.29 04 P -value=o,.003 Rating = 23,90-1,30 hold time To prodict the rating wher hold time is equal to lomin Rating : 23, 30 - 1.30 hold time Predicted rating =23.90-1.30x10 rating 23,90-1 Ratingla.9o 10,90

Extracted text: ) What is the effect on rating of increasing the hold time by 1 minute? g) What is the 95% confidence interval for the coefficient of hold time? f) interpretatio n of Slope the hold time I then the predicted or expected As Increasing rating decreases by -1.30 AS Increasing the hold time by !, then the effertsaa matrny decreases by -130 gJ The 95% confiderce interaval for the Coeficent of minute held te is (-1,9983, -0,6013) 8' + tal2, n-zx SE(B') at a-os ta/2, n-2 =- (-130*0698)18) (- 130 -0698718, - 1.30+0,698718)Ei.99818, -1,998)18, o.601212) to 025,8=2.306 - 1,302,306 X0, 303 (-1,9987,0.013) 2