attached below
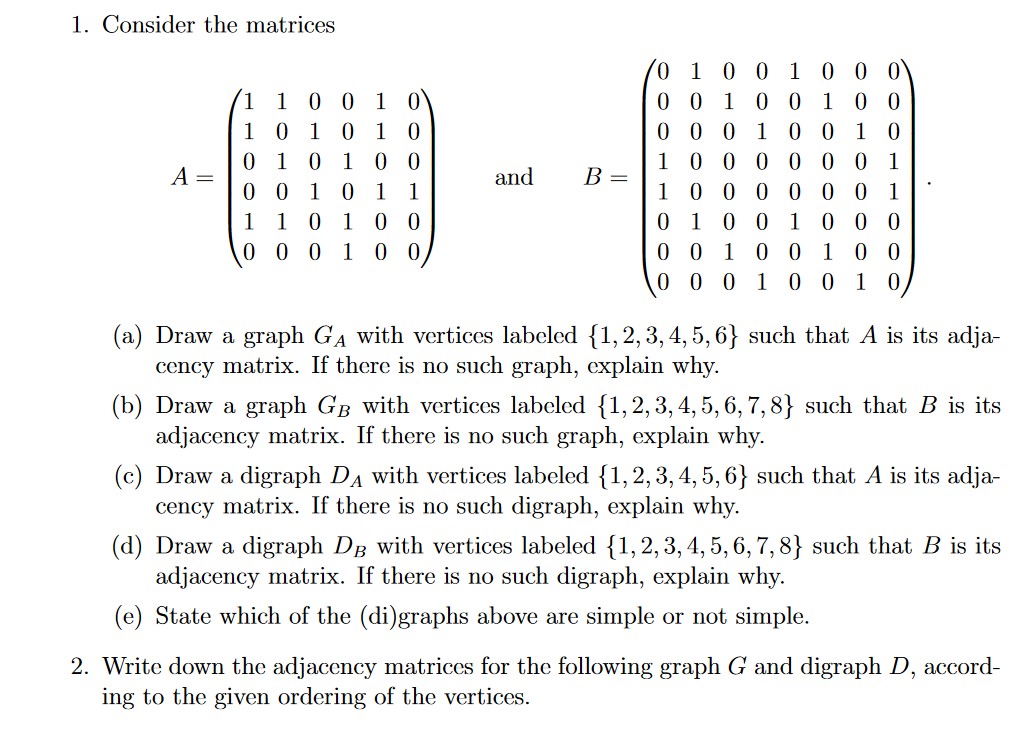
Extracted text: 1. Consider the matrices 0 0 0 0 0 1 0 0 1 0 0 0 0 0 1 0 0 10 1 00 0 00 0 1 0 0 0 0 00 1 0 0 1 0 0 0 1 0 0 1 (1 1 0 0 1 0 10 10 10 0 10 1 00 A = 0 0 10 1 and B = 1 1 1 1 0 0 0 0 0 0 1 0 0 1 1 0 0 1 0 0 0 0 0 100 1 0 0 10 (a) Draw a graph GA with vertices labeled {1, 2, 3, 4, 5, 6} such that A is its adja- cency matrix. If there is no such graph, explain why. (b) Draw a graph GB with vertices labeled {1,2, 3, 4, 5, 6, 7,8} such that B is its adjacency matrix. If there is no such graph, explain why. (c) Draw a digraph DA with vertices labeled {1,2, 3, 4, 5, 6} such that A is its adja- cency matrix. If there is no such digraph, explain why. (d) Draw a digraph DB with vertices labeled {1,2,3, 4, 5, 6, 7,8} such that B is its adjacency matrix. If there is no such digraph, explain why. (e) State which of the (di)graphs above are simple or not simple. 2. Write down the adjacency matrices for the following graph G and digraph D, accord- ing to the given ordering of the vertices.